Introducing Systems of Equations - Exploring Scenarios

http://www.thedogtrainingsecret.com/blog/teaching-dog-tolerate-love-children/ 

I always like to start a new unit with some understanding of why this might be useful and what the underlying idea is for the concept. What really is a system of equations? What does it represent?

Well a system of equations is usually defined as: A system of equations is a collection of two or more equations with a same set of unknowns. (SOS math)

What does that mean? How do we start exploring that? Dan Meyer has an interesting blog post (dy/dan) on this from February 2014 

[Fake World] Culture Beats Curriculum

I recommend reading it and considering how it fits into how you introduce systems. I like his approach and I also like to look at some scenarios that later we will describe algebraically with systems of equations. But right now we just consider them and try solving them without algebra (draw a picture, act it out, educated guess & check, make a table). 

Consider the classic "kids and dogs" problem: 

 In the park one day, I saw a bunch of kids and dogs.  Counting heads, I got twenty-two.  Counting legs, I got sixty-eight.  How many kids were in the park?  

(I bet you are trying to write a system to solve this right now aren't you? stop!!)

You can actually solve this by drawing a picture or more dramatically by acting it out. I saw this years ago in an issue of Mathematics Teacher (NCTM) but do not have the reference to it now. It's so so cool and can be done with younger kids. If you are going to act it out create a problem with less heads because that number of students will have to stand in front of the class.

Drawing a Picture

To draw a picture you draw twenty-two heads. And since you know that both kids and dogs have at least two legs per creature, draw in two legs for each. (I have students do this on the white board - one draws all the heads, then someone fills in the two pairs of legs each). 

How many legs have we drawn so far? 44 legs

How many legs did you originally count? 68

So what does that mean? (there are 24 more legs that we are not seeing yet in our drawing)

Someone will come up with that we have to draw those other legs on our creatures - doing two per creature until we use up all those 24 extra legs. (12 pairs)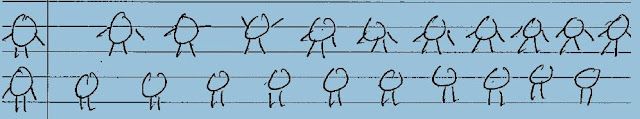

Visually we see 12 creature with 4 legs (dogs) and 10 creatures with 2 legs (kids).

Students love this by the way - it is fun.

Acting It Out
You have the total number of heads acted out by that many students. So with this problem you have 22 students stand in front of the class (really a good idea to scale this down to a smaller number of heads for acting out). Now tell them that some of them are kids and some of them are dogs. But we'll start by saying that all of them will raise their arms (or front legs) in the air. Then we ask rest of the class - how many legs do you see on the ground? (44) But how many legs did we originally count? (68) So how many of those front limbs in the air are actually dog legs? (24) and how many pairs of front legs does a dog have? (2) so 24 legs is how many dogs? (12 dogs!) and from there you can calculate the number of kids (10).

This is a lot of fun too, just hard to do individually or at home. But it's a good way to introduce this and then show them the draw a picture method.

Now students get stuck on wanting to draw a picture but I want them to experience other methods too. You'll find in the next two methods students will really really want to start writing algebraic expressions and equations. I avoid doing much of that, however some little expressions for the conditions can be useful for some of the trickier conditions. But don't do any equations yet!

Educated Guess & Check
Guess and check seems so "random". But with educated guess and check students consider the result of a guess and then adjust in their next guess if the first guess is wrong. Making a table to organie their guesses is a good idea too. Have students consider this problem: 
The sum of Freida & Gene’s ages is 21. Freida’s age is 3 less than twice Gene’s age. How old are both Freida and Gene?
 After some discussion show students how to set up an organized guess & check table with four columns – Freida’s age, Gene’s age, check condition #1, check condition #2. Describe in words, do not get into writing equations (that’s a future lesson). Use the conditions to guess & check, continue until solution is found.

Make a Table

Now you might think - hey they made a table in the above problem. But that was just to organize their guesses. This table is to keep track of a pattern and look for a common result for two items. Consider this plant growth problem:

Plant A and Plant B are on different watering schedules. This affects their rate of growth. You will compare the growth of the two plants to determine when their heights will be the same.

Plant A starts at 5 inches tall and grows 2 inches per week.

Plant B starts at 2 inches tall and grows 3 inches per week.

When are the two plants the same height?

We can start by deciding what the independent and dependent variables are (IV # of weeks, DV total height of plant in inches). And then we can make a set of tables to keep track of the plant growth for each over a number of weeks.

After filling in the tables students can see a common set of values.

Be sure students can articulate what that ordered pair (3, 11) represents!

From these examples I then give them a practice worksheet of problems to work on in their small groups and to complete for homework.