Beautiful Math: Unit 7 Laying the ground work for Properties of Exponents

Sunday, July 26, 2015

Unit 7 Laying the ground work for Properties of Exponents

When we were first starting our curriculum writing for unit 7 we were once again horrified to see that students start with properties of rational exponents. This is in Algebra One. I've always taught the initial properties of exponents with integer exponents. I guess students will now learn this in sixth grade so that by ninth grade they are all set and we can go write into fractional exponents. But my students are very far from that reality (especially since our first common core year in Algebra One was also the first year they did common core in 6th grade in our school system). Yes, students see the properties in Pre-Algebra (8th grade) but have no understanding of where they come from, they just remember there were some rules they memorized.

So back to square one.

I'm a big fan of develop the knowledge, don't memorize rules. That way you can recreate something you may have forgotten the details for as long as you can remember the basics and develop knowledge. My high school years (in the 70s) were spent memorizing formulas and being discouraged from asking why. Very frustrating! So as a high school mathematics teacher I feel it's important to develop those whys.

This blog post is on a discussion day that I have with students in reviewing some properties of mathematics and reminding students of the awesome power of ONE! (who would think ONE would be such a powerful number!).

After the unit test from the previous unit - I jumpstart thinking with this exponents vocabulary puzzle sheet. They complete that for homework.

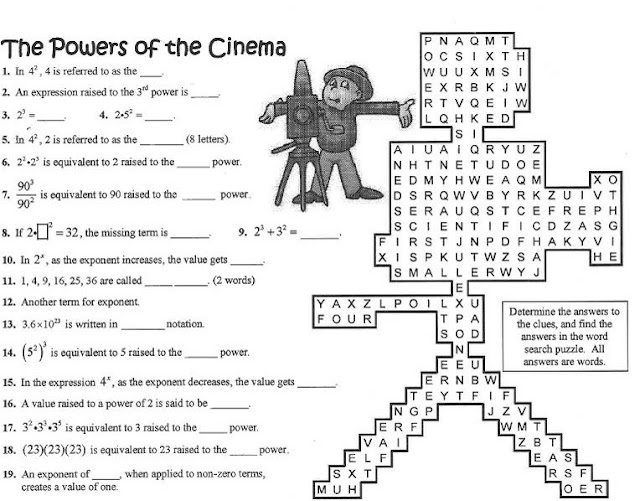
Then I have students do this worksheet as a warm up - all problems they should be able to do from their previous experience with exponents.

So now we are ready for our discussion class. After going over the warm up I refer to the following (written on the board):

· 1) Definition of Exponentiation

· 2) Commutative Property

· 3) Associative Property

· 4) Identity Property of Multiplication

· 5) What's behind the process of simplifying fractions (or why "cancel" is a dirty word)

We talk about what each of these mean. Here's a basic "script" of how our discussions go (I typed it all up one year to have a hard copy). I don't just talk through all these things, I ask lots of leading questions and ask students to give me examples. They should know all this material, they just need a kick start to remember.

1) Definition of Exponentiation

The exponent of a number says how many times to use the number in a multiplication.

In 8² the "2" says to use 8 twice in a multiplication,
so 8² = 8 × 8 = 64

Some more examples:

Example: 5³ = 5 × 5 × 5 = 125

In words: 5³ could be called "5 to the third power", "5 to the power 3" or simply "5 cubed"

Example: x⁴ = x · x · x · x

In words: 2⁴ could be called "2 to the fourth power" or "2 to the power 4" or simply "2 to the 4th"

So, in general:

aⁿ tells you to multiply a by itself,

so there are n of those a's:

2) Commutative Property

The word "commutative" comes from "commute" or "move around", so the Commutative Property is the one that refers to moving stuff around. For addition, the rule is "a + b = b + a"; in numbers, this means 2 + 3 = 3 + 2. For multiplication, the rule is "ab = ba"; in numbers, this means 2×3 = 3×2.

3) Associative Property

The word "associative" comes from "associate" or "group";the Associative Property is the rule that refers to grouping. For addition, the rule is "a + (b + c) = (a + b) + c"; in numbers, this means 2 + (3 + 4) = (2 + 3) + 4. For multiplication, the rule is "a(bc) = (ab)c"; in numbers, this means 2(3×4) = (2×3)4.

4) Identity Property of Multiplication

The identity property of multiplication, also called the multiplication property of one says that the value of a number does not change when that number is multiplied by 1.

5) What’s behind the process of simplifying fractions…(and why you can’t “cancel” when it’s addition)